Toronto/CMEDIA: A longstanding mathematical problem, an “einstein” considered impossible for more than 60 years, until now, was reportedly discovered with the help of an Ontario scientist.
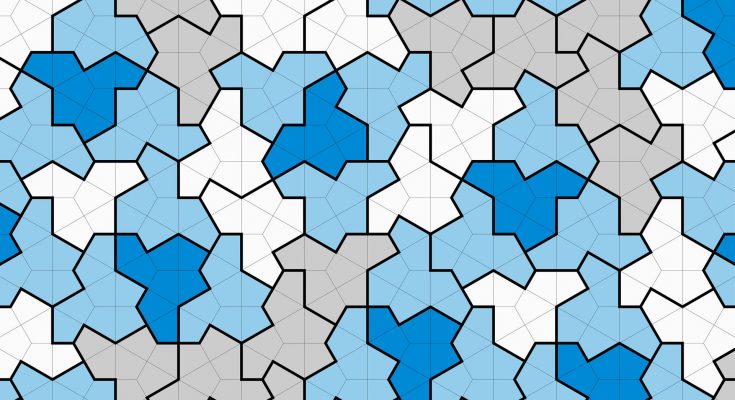
A formation of shapes that perfectly interlocks with one another, without gaps or overlaps, an “einstein” is similar to the bricks that assemble a house.
But in an einstein the pattern of shapes never repeats itself.
Until this month, scientists had yet to discover a shape that could create such a pattern.
“Dave and I had been in touch over the years and we belong to the same old-fashioned listserv for people interested in tiling, a mix of tiling enthusiasts, programmers and mathematicians,” recalls Cheriton School of Computer Science professor Craig S. Kaplan, who collaborated with Smith, software developer Joseph Myers and mathematician Chaim Goodman-Strauss on the paper that has proven that the elusive einstein exists. University of Waterloo news reports said.
Visually resembling eight kites glued together to make a single 13-sided shape, Kaplan and his colleagues have called that shape “the hat” because of its resemblance to a fedora.
The existence of an “aperiodic monotile” was put forward by the mathematician Hao Wang in 1961.
Soon after,one of Wang’s students reportedly discovered an aperiodic set of 20,426 tiles, and then, a set of 104.
Roger Penrose, mathematical physicist and Nobel Prize winner reduced that number in the 1970s to two.
But over the last several decades, progress has remained standstill.
It was only after receiving an email last November from David Smith, a retired printing technician in England, that Kaplan became aware of the existence of “the hat”.
“He emailed me and asked if the work I had done previously might be useful in trying to understand its properties,” Kaplan was reported recalling
Being interested in previously crafted software by Kaplan capable of automating a visualization of hundreds of hats glued together, Smith wanted to use Kaplan’s software to find out if this shape could solve the longstanding problem.
Until this point, Smith had been in pursuit to see if his “hat” could in fact solve the unsolvable.
Within just five months of connecting, a research paper was published by Smith, Kaplan and two others, Chaim Goodman-Strauss and Joseph Myerson on the previously impossible “aperiodic monotile” in Cornell University’s archive awaiting a peer-review.
“It settles a longstanding open problem in mathematics,” Kaplan was reported saying.
#Ontario; #OntarioScientist; #aneinstein; #CraigKaplan